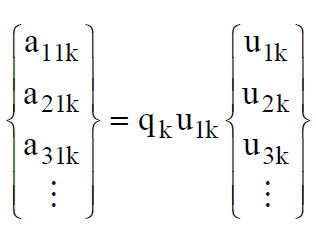Modal Space - In Our Own Little World
How do you select the reference location for a modal test? What needs to be considered? Let's discuss this to see how to think about this.
Now the selection of the reference location is one of the more important steps of performing an experimental modal test. If the reference(s) are selected poorly, then there is a strong possibility that one or more modes of the system may be represented poorly or, in the worst case, not at all. Many times the references are selected with a priori knowledge if similar structures have been tested many times in the past. In these cases, the selection is much easier. But when the structure is unique and has no previous history, then the selection of the reference can be much more complicated. Obviously, experience is a very strong plus in these situations. And it may be that there is an analytical model that may assist in this selection of the reference. So let’s discuss some basics and describe some things to consider when selecting the reference location(s).
The first thing to really show is the basic equation that dominates the selection of the reference. As I always say to all my students, “Remember … the most important answer to almost all of your modal questions is very simply ui uj”. Of course the students all make fun of me for saying this over and over but then they realize that most of their modal questions are often answered with this very statement! So what do I mean by this. Recall that the residue matrix is given by
But we do not collect data for all of these input output combinations (and theory tells us that we do not need to measure all of them either). So there needs to be a very careful selection of which rows or columns are measured. If we look at one column then we can write
Obviously, the value of the mode shape at the reference location must be significant for all the modes to be measured. If this is done then the FRFs measured will have strong response of the modes of the system. But if the value of the mode shape at the reference location is not significant for one or more modes of the system, then the FRFs may not contain strong response for all the modes of the system. This will make the modal parameter estimation process more difficult.
So if an analytical model is available, then the mode shapes can be reviewed to select optimal reference locations. One simple tool that is often used is the drive point residue. Basically, this is an assessment of the mode shape represented as a residue
This is a common tool used in preliminary assessment usually called a Pre-Test Analysis. Of course there are other tools such as Mode Shape Summation, MODMAC, Effective Independence, along with others that are beyond what can be discussed here. But what if a finite element model is not available or (let me say this quietly) what if the model is not correct. So we need to be able to select the references without any previous knowledge or assistance from an analytical model.
So often times, an experimental test is setup and the first thing that is done is to make sample measurements to determine how many modes might exist in the structure. At times, the drive point FRFs are reviewed – possibly the imaginary part of the FRF is viewed. Unfortunately, this is probably not one of the better measurements to view. This is because closely spaced modes may be difficult to identify since all of the peaks of the imaginary part of the FRF will have the same positive or negative going peaks in the function. Actually the non-drive point measurements are better because the values of the amplitude can be both positive and negative enabling a better chance to identify closely spaced modes. For example, two measurements are shown in Figure 1. The upper trace is a drive point FRF and it is impossible to identify that there are two modes at the first peak. The lower trace is a cross measurement and it is more obvious that there are two modes at that frequency. So you can see that a drive point measurement is useful but may also be deceiving in that the strength of each mode is not apparent in the measurement.
As a modal test is set up, often, a random sampling of FRFs is made with an educated guess as to what might be reasonable references. This random selection is shown in Figure 2 with the selected measurements shown in different colors.
The FRFs are reviewed and identification of peaks in the FRFs are noted from one measurement to the next. If all the peaks are the same and no additional peaks are obtained, then the references might be reasonably selected from those measurements made. Unfortunately all the measurements are made in a somewhat random fashion. There is also a very strong possibility that critical modes may be missed with this procedure. (I have seen even the best of test engineers occasionally miss major modes of a structure)
Another possibility to identify potential references is to obtain a small set of FRFs at all of the potenetial candidate reference locations. This set of FRFs is shown schematically in Figure 3. An SVD is then performed on this matrix. By evaluating the SVD of submatrices of this original matrix (ie, removing individual references in a controlled fashion), an evaluation of the number of significant modes can be determined. If the same number of significant modes are obtained, then the reference removed was not a critical reference for the identification of the modes of the system. However, if fewer significant modes are identified then the reference removed was an important reference for those modes no longer observed and should be retained as a reference for the modal test.
So while it is common practice to take a handful of randomly selected FRFs to identify a potential reference location, a possible alternate approach that utilizes a mathematical approach to perform an SVD with a set of FRFs may be a much more rigorous mechanism for identifying potential references. This approach, commonly called the Test Reference Identification Procedure (TRIP), offers a technique for reference determination. This is especially useful when no analytical model is available or when there is skepticism as to the accuracy of the finite element model used for the Pre-Test analysis.
The real trick here is to pick a reasonable ui uj term such that the value of the mode shape at the reference location is a significant value. This will then cause the FRFs to have significant peaks that allow for adequate measurements to be made. Of course, you have to have an idea of what the mode shapes of the system are in order to achieve this. A finite element model or apriori knowledge is very beneficial to accomplish this.
If you have any more questions on modal analysis, just ask me.
Join Pete Avitabile’s Modal Measurement Seminar in Seattle, WA from October 8-9, 2019.